Introduction
Cyclic Steam Injection is a commonly applied in Venezuela to produce heavy oil. The operations consists on injecting a given volume of steam to heat the near wellbore (rock matrix in radius about 40 ft) to reduce the viscosity of the oil which improves the productivity index and increases the oil production rate.
Once the well starts on production, the fluids coming from the unheated zone (at reservoir initial temperature) cool down by convection effects the heated zone (rock at steam temperatures). This cooling process has a damping behavior which is reflected in oil production history.
From the reservoir engineering point of view, thermal simulators are suitable tools to study CSI wells, since energy and flow equations are properly considered in these programs. The system of coupled differential equations are solved numerically by gridding the reservoir, including the corresponding constrains (no flow or aquifer inflow) at reservoir limits and fixing pressure at bottom hole as sink cells. For reservoir engineers; this approach is close enough to real because they are looking for phase distributions and pressures across the whole reservoir along the operational life. Simulators consume computational time and require efforts and expertise for defining the grids and introduce the parameters from the static or geological model, besides.
For production engineers is important to know the well (local) rather than the reservoir (whole area) behavior, then reservoir simulators outputs are references for evaluating, sizing and simulating the artificial lift completions, considering the short term variations in the productivity index and other production conditions such as: temperature, WOR, viscosity, etc. For a production engineer is relevant to predict when those conditions would occur in order to adjust or change the production system and increase the oil rate. Prediction of events is dificult because the cooling down process depends on the rate, which depends on the artificial lift performance. So it is clear that a coupling of reservoir and artificial lift system is required.
The coupling of the artificial lift simulators with a reservoir simulator would be ideal but would take long run times, so for the the same objective but for shorter run time an simplified reservoir model is required. Boberg & Lantz´s simplified analytical model is less accurate than simulators but it is useful because provides easy and quick answers to get an initial idea of magnitudes and behaviors of CSI wells. This analytical model has the same assumption of constant bottom hole flowing pressure as the simulators. Under this assumption all the artificial lift methods are equal which is useless for the production engineer goal of selecting the artificial lift method.
In this work would be shown how to modify the Boberg & Lantz´s model to take into account the effects of the artificial lift methods. Through this approach four cases corresponding to two reservoirs with vertical and horizontal wells were analyzed under different artificial lift systems resulting in four different recommendations.
Theoretical Background
In this section will be discussed different artificial methods and their advantages, limitations, relevant issues and special considerations for handling high temperatures associated with steam. Finally the models for heat losses along the tubing during injection, the procedure for calculating the heated radius and the original Boberg&Lantz´s model will be detailed.
2.1. Artificial Lift Considerations
Any artificial lift method for a CSI wells deals with the following conditions: Initial high temperatures [500 F, 600 F], high initial water cut, high declination of the temperature and productivity index.
Sucker Rod Pumping (SRP) is the most used artificial lift method when steam is injected in both continuos and cyclic schemes, since it supports up to 660 F. This method can handle up to 800 BPD. For higher rates big or special bean units are required, which increases the production cost per barrel.
Continuos Gas Lift (CGL) is also used for steam wells, spring valves must replace nitrogen charge valve. Even for the spring valves, the rubber seals can support up to 500 F.
Intermittent Gas Lift (IGL) could be used for steam wells, but again spring pilot valves must be used below 500 F. Rates should be lower than 300 BPD.
Gas Chamber Pumps (GCP) were used in eastern Venezuela for CSI, in shallow wells where sucker rod pumping gas lock problems happened and high-pressure gas surface facilities were available. There is no restriction for temperatures since only check valves are required down hole.
Jet Pump (JP) experiences with steam applications have been reported in Canada. Temperature restriction concerns with the Pump internal seals, special materials can handle up to 500 F. Notice that the power fluid can reduce the temperature at the pump, which allows higher temperature for the fluids coming from the well.
Progresing Cavity pump (PCP) commercial elastomers can handle only 300 F, which restricts the application when the temperature has declined below this value. A metal rotor can handle high temperatures but for low viscosities (high temperatures) has low efficiency and has poor hydraulic seal because of the high stator-rotor clearance.
Electrical Submergible Pumps (ESP) has temperature restriction for the motor, whose internal temperature cannot exceed 450 F, which means that the formation fluid, acting as cooling fluid, temperature must be lower than 350F. Besides, the seal section life would be shorter.
Heat losses considerations
Steam injection efficiency is affected by heat losses along the well from the wellhead down to the perforations. Heat isolators are used to reduce those losses, some times just the gas in the annular is the isolator. Usually operators know the thermal energy injected at the wellhead by measuring the temperature, steam mass flow, and steam quality at the surface but not at down hole. It is clear the importance to calculate heat losses in order to determine the ultimate thermal energy injected to the pay zone because this value affects the radius of the heated zone and future productivity index. The equation for the total thermal resistance is:
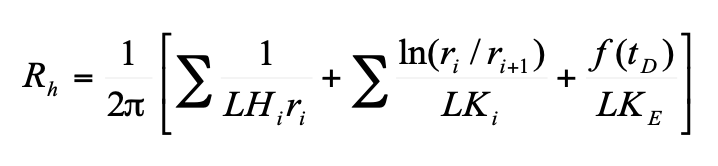
In this equation1 there is a time dependent f(tD) term because the overburden surrounding along the well is heated. As expected there are terms concerning heat transfer coefficients (Hi) and thermal conductivities (Ki) for the tubing, casing and cement materials. The heat losses rate are given by the temperature of the steam minus the average temperature along the well and then divided by the thermal resistance
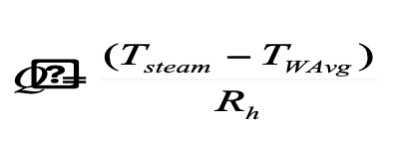
Heated Zone
Marx-Langenheim2 solution to determine the radius of the heated zone was selected. This solution accounts for the losses to the under and over burden layers. The bigger the radius the bigger the losses until no more growing is possible, this condition happen when heat losses to the under and over burden layers reaches the heat injection rate from the bottom of the well bore into the pay zone.
Marx-Langenheim solution involves the complementary error function (erfc) evaluated at dimensionless time (tD) which depends on reservoir thickness, the thermal rock properties of the reservoir (Mr) and under and overburden (Ms, αs).
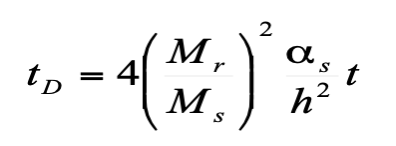
The heated area is given by

Boberg & Lantz Model
Boberg&Lantz3 analytical model provides important values for a given temperature: the Productivity Index, expected rate, and the expected new temperature; therefore the temperature and rate profile vs. time can be determined by iteration.
The Boberg and Lantz solution represents the convection cooling processes for a single Cyclic Steam Injection. The productivity index correspond to a simple model of a heated radius with high temperature then a temperature drop (step function with no transition zone) down to the original “cold” temperature (Fig.1). By splitting the total pressure drop in the cold zone pressure drop (from the drainage radius to the heated radius) and the hot zone pressure drop (from the heated radius to the well radius) in terms of the Darcy law:
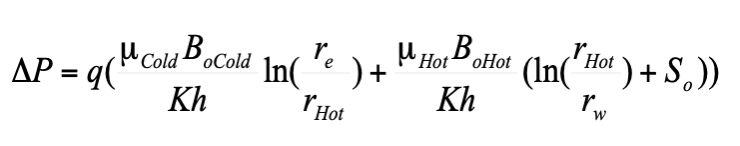
Where So is the original total skin without heat (damage and pseudo skin for perforations, etc.),. Considering that the temperature variations in Bo are neglectable compared with viscosities variations then the productivity index can be expressed as:
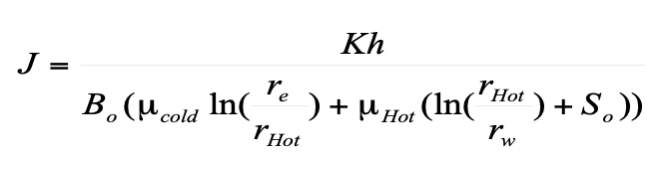
Boberg and Lantz assumed that the bottom hole flowing pressure never changes, then a ratio between the cold and heat rates can be determined.
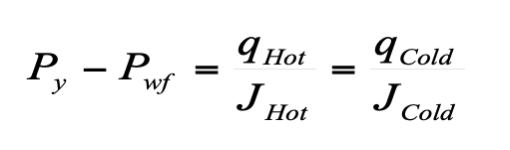
In this case Boberg and Lantz´s expresión for the expected rate is given by:

In this expression Qcold is given by production history of the well under study.
For the new expected temperature the amount of heat transported by the oil and water being produced must be calculated using the specific heat of oil and water. Then a cumulative dimensionless heat produced (fpD) is defined as the ratio between cumulative heat produced and the total heat injected.

The Boberg&Lantz solution takes into account the conduction heat losses in reservoir (horizontal to the unheated) and for the surroundings (vertical) through special functions (fHD, fVD) evaluated at corresponding dimensionless time (tHD, tVD). The heated zone radius is assumed constant. The analytical expression for the new average temperature for the heated zone is:
![]()
Knowing the new average temperature, then new viscosity, productivity index and rates can be determined and the whole procedure is repeated until the original reservoir temperature is reached.
Boberg established and used eq.6, it is clear that cyclic steam injection is a thermal stimulation because of the oil rate increase but and additional comment should be point out by rearranging the previous equation.
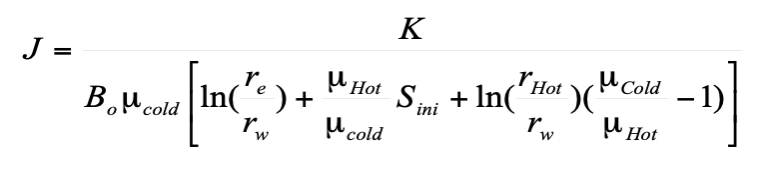
By definition the new skin S with heat is

This equation shows two important facts: the original skin effect near well bore is reduced by the viscosities ratio, and the increase in production depends on the heated radius and the viscosities ratio.
Approach
In the following section, the modification of the Boberg & Lantz´s approach considering the effects of the artificial lift methods will be detailed.
It is well known and physically expected and that the bottom hole flowing pressure is not a constant value because of the great changes in productivity index, this fact is confirmed by production profiles. Then Boberg and Lantz model is acceptable for productivity indexes and cooling process but not suitable for the expected rate. Having a better value for the expected rate then the cooling process accuracy would improve, for this purpose nodal analysis have to be applied.
Nodal Analysis
Knowing the productivity index J, the inflow performance relationship (IPR) curve is given by a simple model, for example Vogel’s curve.
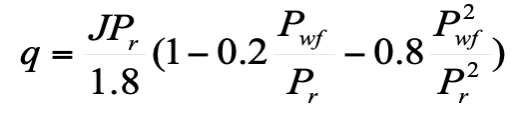
Since the temperature is continuously decreasing as cumulative oil production increases, then the absolute open flow (Qmax) is also decreasing showing the detrimental of the IPR performance curve (Fig.1).
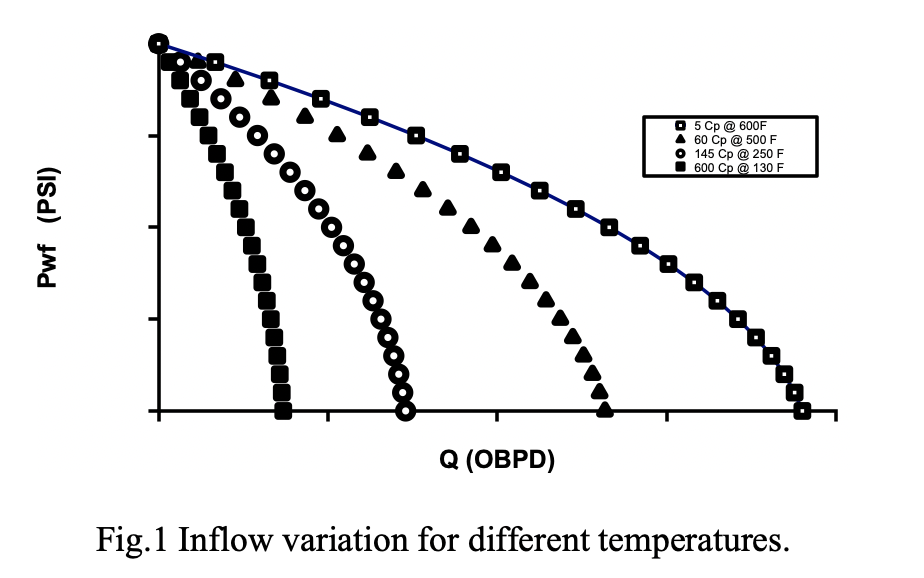
Not only the inflow changes with time but also artificial lift performance (outflow curve) changes continuously usually in detrimental (Fig.2), but not always because for example PCP Metal-Metal would improve as temperature decreases because viscosity increases producing a better hydraulic seal.
Generating numerous outflows for different viscosities would require multiple simulations, which means a huge effort and it is CPU consuming.

A new idea was employed in this work, first step is to generate outflows for some preselected viscosities μo (1, 5, 50, 100, 600 cp) and some preselected rates qo as usual in nodal analysis, and then generate the outflow for any required viscosity using the following interpolation.
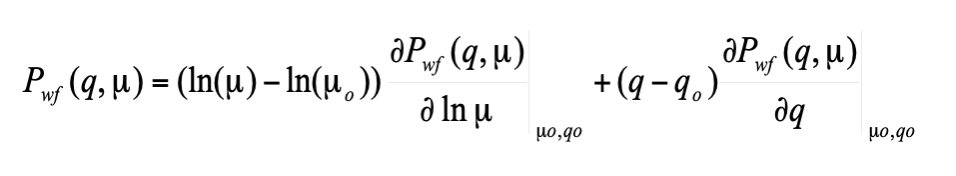
This procedure must be done for each artificial lift method with the corresponding simulator. Although, open code source was used in this work, should be notice that this procedure makes possible to avoid program coding and allows using artificial lift commercial software.
Finally, intercepting the corresponding inflow and outflow curves, for the viscosity under consideration, a better rate estimation is obtained. It is clear the importance of coupling Boberg & Lantz model with nodal analysis, because nodal analysis just gives the real rate but cannot predict when that viscosity associated with the current temperature will occur. Notice that even the rate previous to the injection (cold state) can be determined, so no production history is necessary at it is in the original Boberg&Lantz model (Eq.7).
Results
The original goal of this project was to determine the best artificial lift method for producing CSI wells in two heavy oil reservoirs (designated A and B) in off shore Lake Maracaibo shallow waters. Completions with pumps and gas lift mandrels were analyzed in order to evaluate change in the artificial lift method during the cooling period. The rate and the total heat injected are the same for all the cases in order to have a better comparison among the production methods.
Both reservoirs have similar permeabilities, and fluid properties but different depths, which reflects as higher temperature and lower viscosities for the deeper one (reservoir B); therefore, the productivity index and production rates are higher in this reservoir. In order to increase the rates the shallower (reservoir A) is commonly produced by cyclic steam injection, most of the wells are verticals and few horizontals.
Case 1. Reservoir A with Vertical well
Continuos gas lift and CSI is the most common application in this area, it is a very important case since it provides production profiles and history information for matching the proposed approach. Permeabilities and other petrophysical data is obtained through standard test (well logs, build ups, cores, etc) but thermal properties of the rock (reservoir, under and over burden) must be tuned by running the model and try to match the shape of the oil rate profile vs. time. Thermal conductivity and diffusivity are key values to control the peak and the cooling time. Figure 3 shows the productivity and temperature profile vs. Time for different production methods.
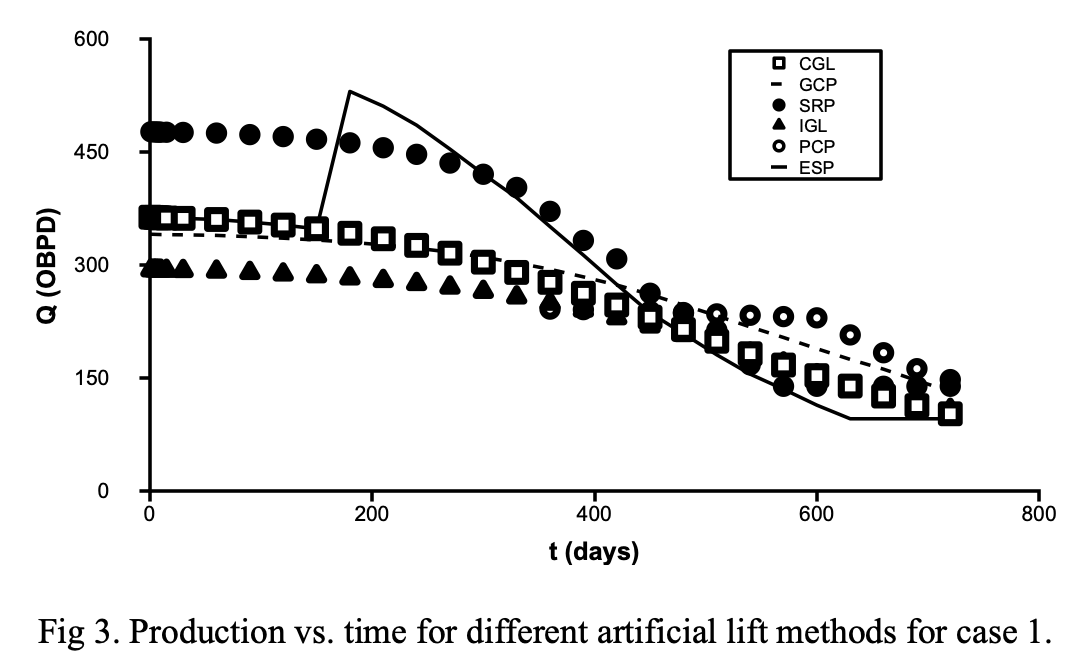
Temperature changes from 580 oF to 130 oF (Fig. 4), then the viscosity reduces from 600 cp down to 5 cp. In this case gas lift is the best option even though Sucker Rod pumping can handle more production for hot and cold conditions, this explains why is so popular in on shore thermal applications, but this is an off shore application and the available platforms at the well are too small for installing the beam unit.
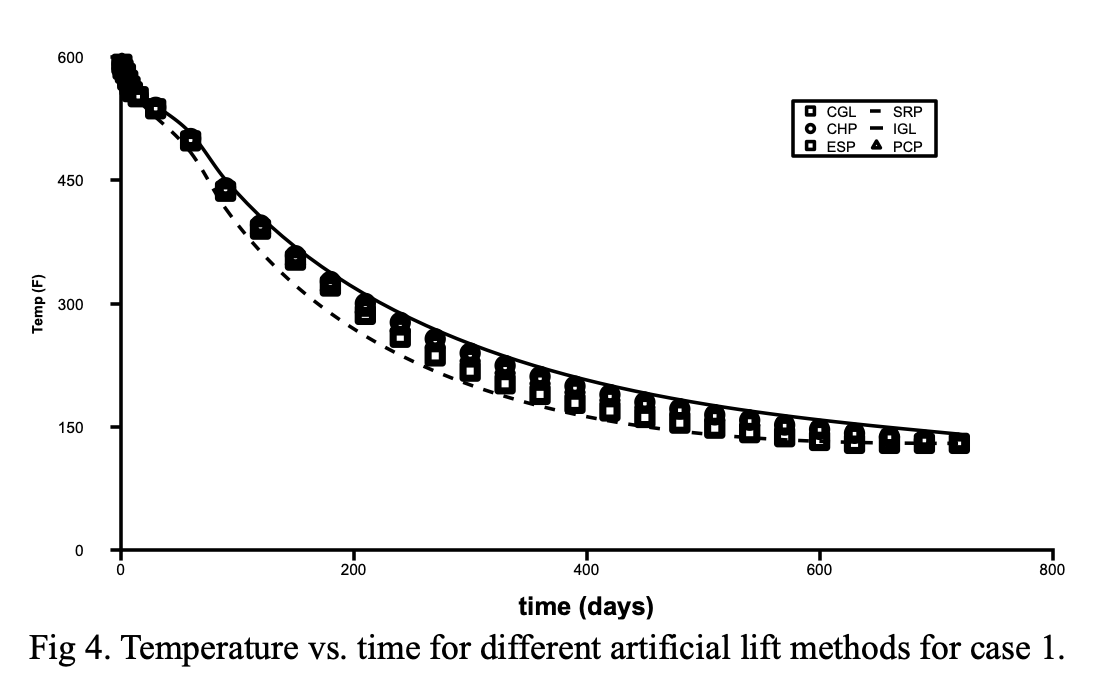
Notice that ESP initial peak is the highest but occurs at day 180, because after this time the temperature is below 350 F allowing starting the motor, which means that at the beginning gas lift must be used. Same happens with PCP but later (day 350) because temperature must be lower 220 F.
Another interesting quotes are that ESP also cools down faster because of the higher rates and has the lowest rate for cool production because pump performance falls dramatically as the viscosity increases.
Case 2. Reservoir A with Horizontal well
Few horizontal wells in this area but all of them using continuos gas lift and Cyclic Steam Injection. The thermal properties determined in case 1 were used for the remaining cases, matching the production profile for horizontal wells.
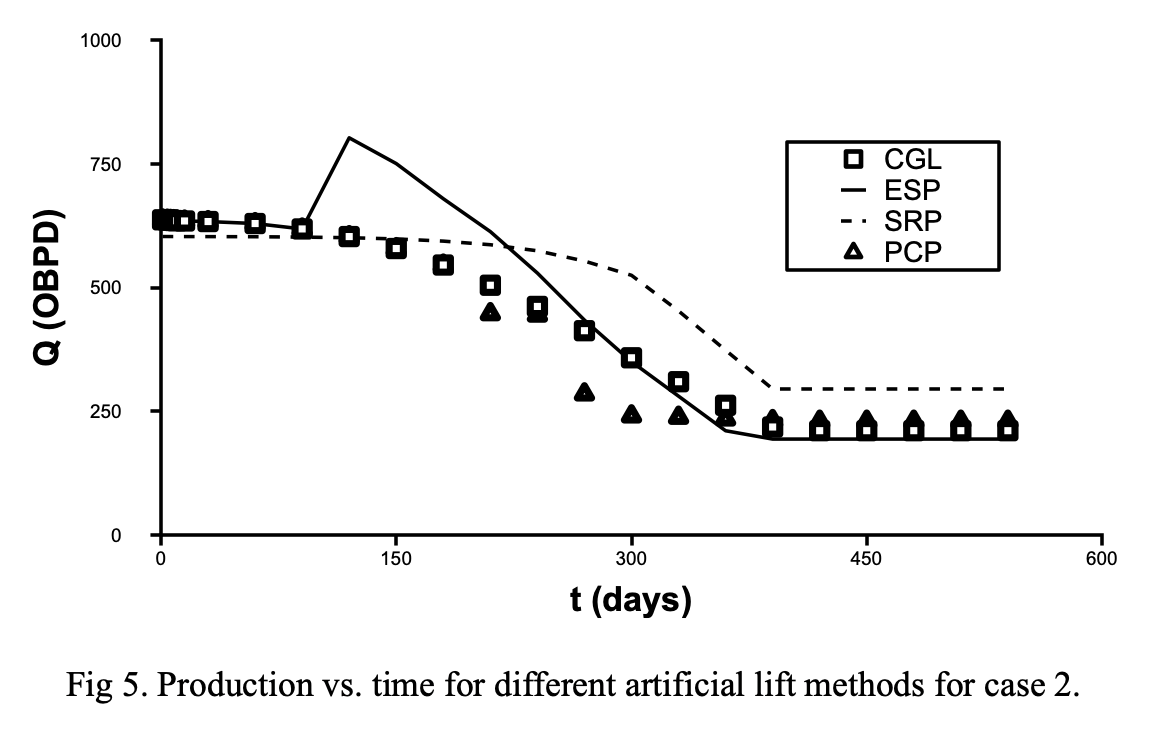
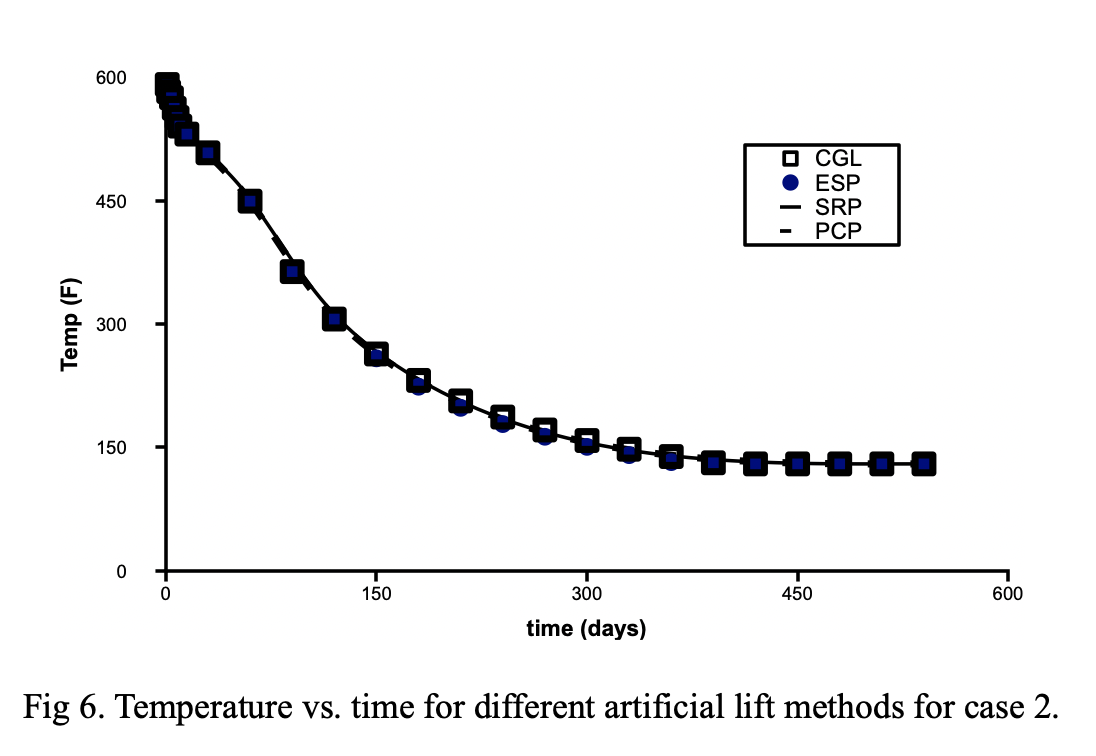
Joshi productivity index was used together with the Skin expression (Eq.12). As expected, production and temperature profiles changed (Figs. 5-6).
For this case at the beginning of cycle, continuos gas lift is is similar to sucker rod pumping, in contrast with the case A where there is a difference. Intermittent gas lift was not considered because of the high rates associated with a horizontal well.
Case 3. Reservoir B with Vertical well
Since CSI has not been applied in this reservoir then the thermal properties were assume the same as A, which has sense because they are in the same area but different depths. Since reservoir original temperature is 145 F, then the viscosities reduces from 96 to 2 cp that is the reason for the smaller oil rate increase compared with case 1. Besides the deeper the reservoir the bigger the injection heat losses along the well.
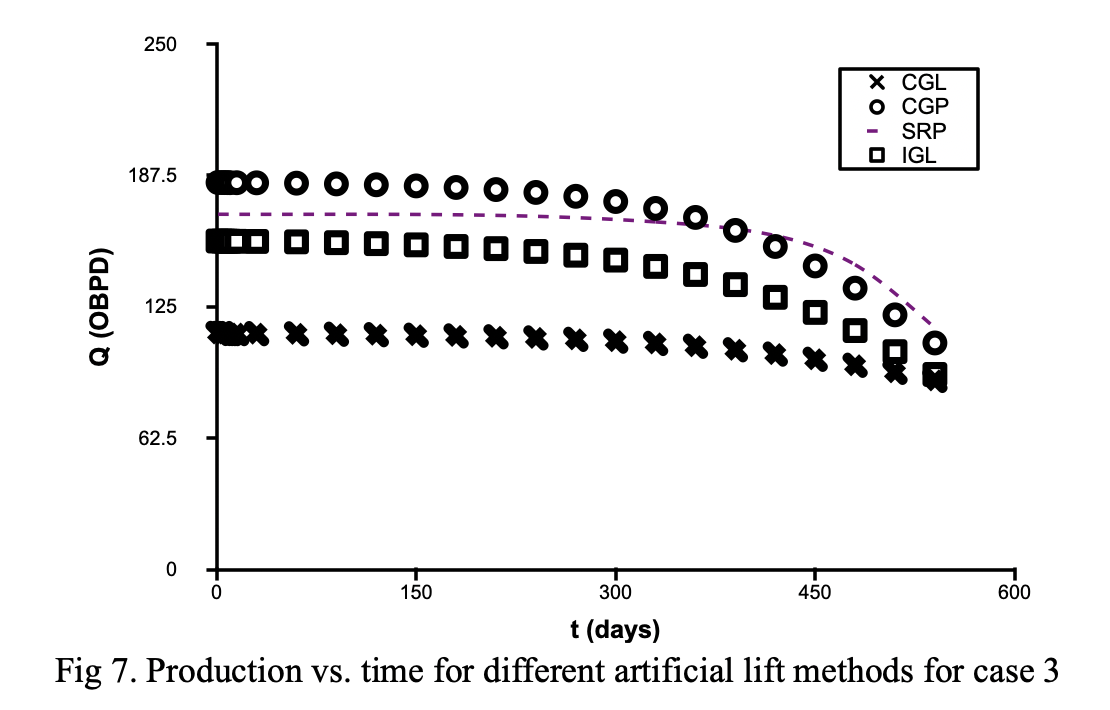
Notice that low production methods, such as intermittent gas lift, are analyzed. Gas chamber pumps have the best performance.
Case 4. Reservoir B with Horizontal well
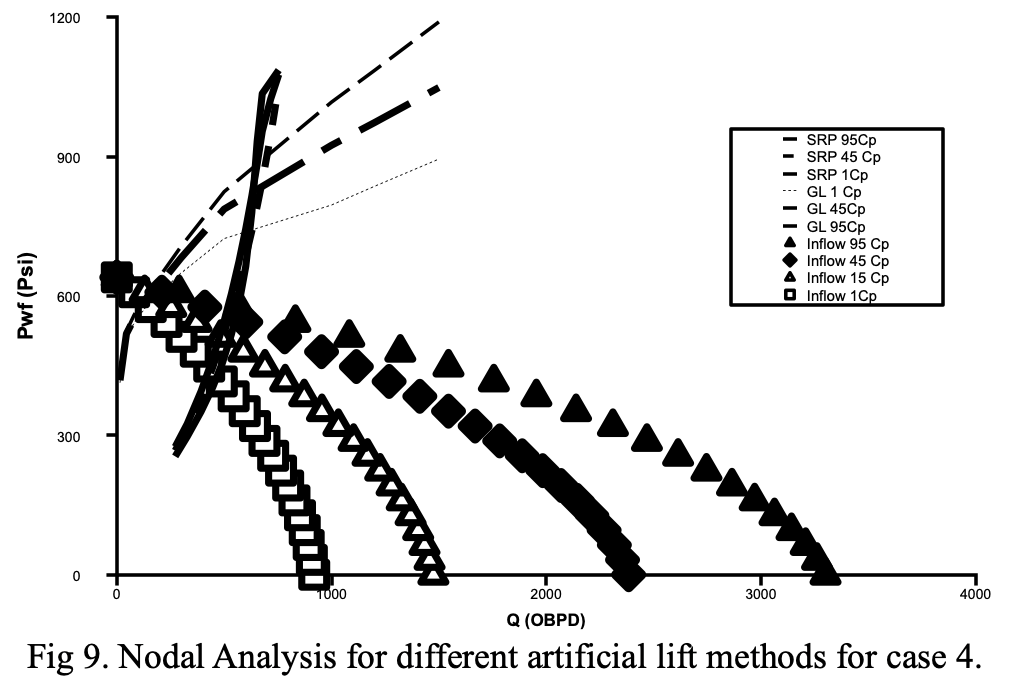
Probably this is the most interesting case since the production profiles shows an unexpected result (Fig. 8). Sucker Rod pumping is the best method but, should be noticed that it produces more oil than the others methods even in cold production. The reason for this behavior is explained by nodal analysis (Fig. 9), it indicates that the reservoir inflow potential is huge for different temperatures and the considered methods do not have the capacity to lift the fluids. ESP cannot be applied because GOR is too high.
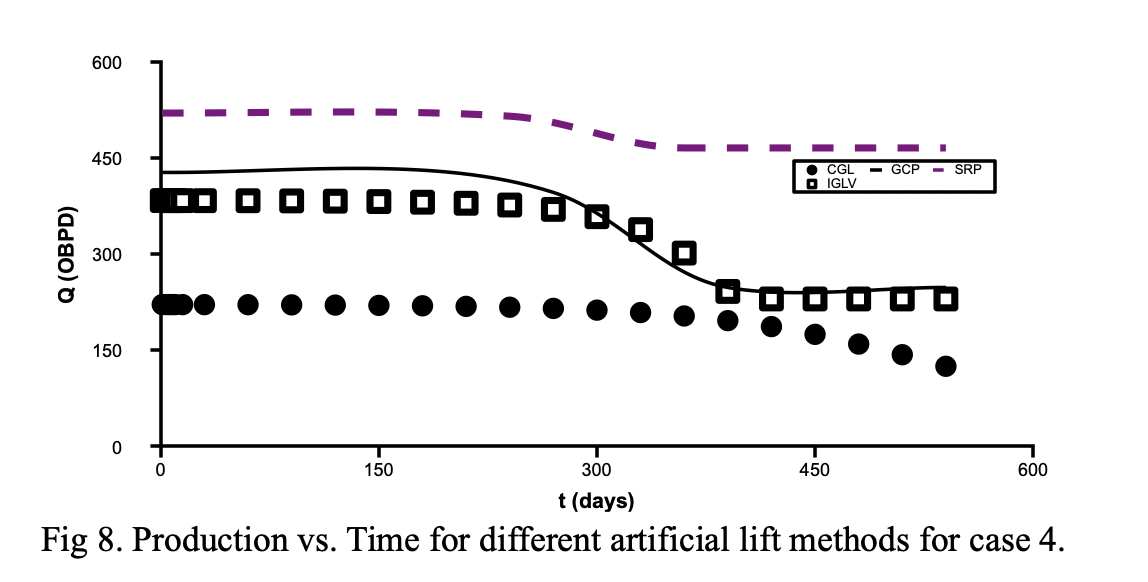
This case shows the potential of this approach since this unexpected situation cannot be detected by the Boberg &Lantz original approach. Besides, it indicates that there is a marketing opportunity for developing a new technology pump able to handle high rates, high temperatures and high GOR. Such technology would allow intercepting the inflow curves in the low flowing pressure area (200 PSI).
Conclusions and Recommendations
The coupling of simple reservoir and artificial lift models for Cyclic Steam Injection wells improves the results accuracy in the production profile allowing better analysis and shows opportunities or ideas for other research projects. Therefore, based in the proposed approach and results it is recommended to couple a thermal reservoir simulator with artificial lift simulators for different production methods.
The suitable artificial lift method for Cyclic Steam Injection wells not only depends on the temperature constrains but also on other conditions such as: pressure, GOR, Productivity index range (cold & hot), depth, etc.
Since a better artificial lift performance reduces the cooling period, then the injection parameters such as: Total effective heat injected, effective heat injection rate, etc., must be adjusted in order to maximize rate or economical indicators.
Economical analysis should be performed considering steam injection costs and artificial lift operational cost and expected life under extreme operational temperatures.
References
PRATS, M., SPE “Thermal Recovery Monograph” 1972.
MARX, J.W. AND LANGENHEIM R.H. “Reservoir Heating by Hot Fluid Injection” Trans. AIME (1959), 216, 312-315.
BOBERG, T.C. AND LANTZ, R.B.: “Calculation of the Production Rate of a Thermally Stimulated Well”, J. Pet. Tech. (Dec.1966), 1613-1623.
VOGEL, J.V, SPE 1470 “Inflow Performance Relationships for Solution Gas Drive Wells”, SPE 41 Annual Fall Meeting, Dallas, Oct 2-5, 1967.

